Nth Octave Bands
Calculating the Mid and Corner Frequencies
The mid and corner frequencies of nth octave bands are calculated as mathematical rows. For example, as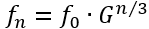 
for the mid frequencies of the 3rd octaves. The row is centered at the
reference frequency f0 = 1000 Hz
The regulation IEC 61260 defines the octave frequency ratio with
G = 103/10 ≈ 1.99526
meaning that the frequency increases with a factor of 1.99526 with each octave step. Filters designed on this definition are called base-10 filters. For pratical reasons, some filters have been designed based on a doubling of the frequency with each octave (G=2). The deviation between these base-2 filters and the base-10 filters increases with the difference between the mid-band frequency and the reference frequency.
N-th octave frequencies for odd dividers:
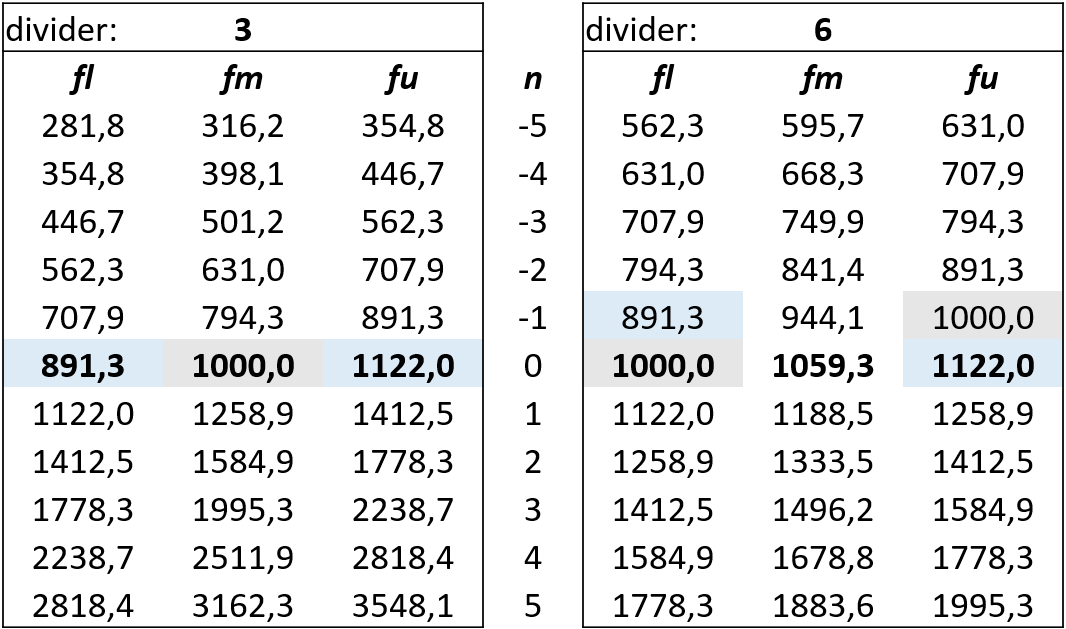
To align two 1/6 octaves within one 1/3 octave band, for example, the rows of the frequencies with even dividers are shifted by n/2:
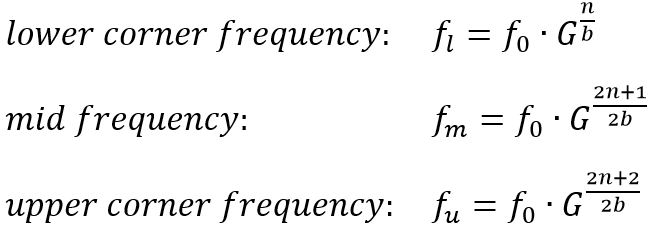
The corner frequencies of the even dividers match the mid frequencies of the odd dividers
Changes from PAK 5.x to PAK 6.0
With the introduction of PAK 6.0 the octave ratio was changed from base-2 to base-10. Therefore, the frequency bands are slightly shifted. The difference is mainly less than one percent and biggest at very low and very high frequencies. The exact value for the 4 Hz mid frequency, for example, changed from 3.906 Hz to 3.981 Hz.
With PAK 6.0 the frequencies for the even dividers are now calculated as described above, according to the regulation. In previous versions they have been calculated with the same formulas as for the odd dividers (in order to fulfill customer request for same mid frequencies for both odd and even dividers).